MODELLING CONCEPTS
Topology.
Topology and geometry are two often confused terms.
Geometry (in 3d) is considered to be simply the points, edges and faces which make up an object. It is often used as a way to describe the "Weight" of an object. That is how many vertices, edges or faces it contains. The word "Geometry" is not often used outside of this context.
Topology is the understanding of the way this geometry is connected. Good topology has flow. bad topology does not. The "weight" of topology is irrelevant as this will be controlled by the subdivision surface modifier.
The topology of a subdivision surface is thought to have a defined logical structure in the way that polygonally modelled objects do not.
Subdivision surface models can be thought of as many areas (or local geometries) bounded by continuous loops, connected together in a logical formation.
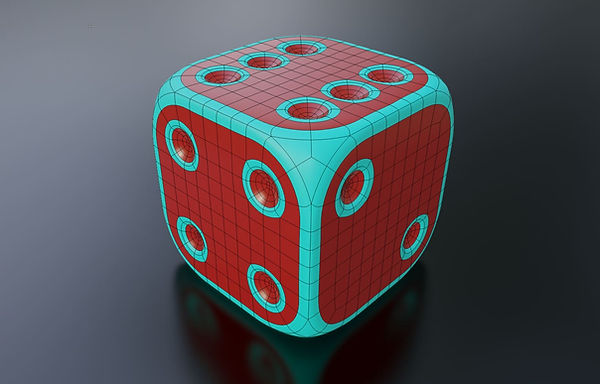
Using the dice below, all of the red areas are separate local geometries and the blue areas are the boundaries connecting them. The blue areas can have loops inserted between their edges which will directly control the curvature over the edge regions without needing to worry about bevels or additional geometry which would immediately bind the curvature to a set resolution.
It is often useful, for simple objects, to think about them as having a top, a bottom, a cross section and areas of curvature connecting these things. The basis for this can be seen in its simplest form when we make the Cylinder as a subdivision surface model.
For more complex objects it is best to consider each local geometry (The red areas in the dice) as having a shape and an ability to connect to other meshes through the addition of a boundary or by substituting the tops and bottoms of objects with entire additional subdivision surfaces.
The structure and vertex count of each local geometry do not necessarily matter as the boundary areas will be used to convert between various vertex counts using connection reduction techniques. (See Radial Connection Reduction)
Local Geometries (Red) and Boundary regions (Blue)